The Value of Uncertainty
- Mikael Palmblad

- Oct 13, 2021
- 7 min read
Updated: Nov 30, 2021
Is there any value in uncertainty? Do you know uncertainty? Is uncertainty a friend, or a foe? Let's start by asking another question: What is uncertainty?
First of all, uncertainty stretches wide in the spectra that it covers. On one side there is guesswork, which means that you don't know anything. On the other there is perfect knowledge, i.e what you say will happen happens. In between there's a continuum, going from zero uncertainty to perfect knowledge. And with that, can you answer the question: Is there any value in uncertainty?
Unfortunately, the answer is not black or white, but rather the value in uncertainty depends on the alternative. If the alternative is perfect knowledge, then uncertainty is actually a liability, posing a threat to a decision of not fulfilling its desired outcome. However, if the alternative is guesswork, then uncertainty definitely has a value. The only question is, what do you do with that value?
The Five Orders of Ignorance
If you want to understand what to do with uncertainty, you can look at uncertainty according to the five orders of ignorance, put forth by Phillip G. Armour:
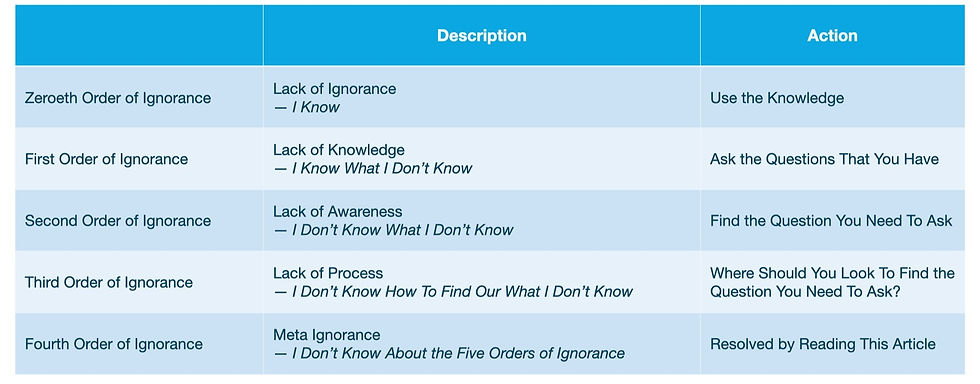
- Zeroeth Order of Ignorance At the top, you have the zeroeth order of ignorance, which can be described as a lack of ignorance. That is to say I know, and what you can do then is to actually use that knowledge that you know to our advantage. - First Order of Ignorance The first order of ignorance is a lack of knowledge, I know what I don't know. You do, however, have a question that you need an answer to. With formulating this question and actually asking it, you can now pinpoint what you need to know and fill that gap with knowledge.
- Second Order of Ignorance The second order of ignorance can be called lack of awareness, I don't know what I don't know. The problem here is that you don’t know how to formulate the question that needs asking. Thus, the problem here is to understand what question needs asking.
- Third Order of Ignorance The third order of ignorance can be called lack of process. I don't know how to find out what I don't know. At the stage, you're lacking in method of how to look for your question that needs formulating in order to be asked.
- Fourth Order of Ignorance At the fourth order of ignorance, the ignorance becomes a bit meta, as it’s about being oblivious to the existence of the model at all, I don't know about the five orders of ignorance. Conveniently enough, just by reading this article, you have now left the fourth order of ignorance.
Subsequently, this is uncertainty as we know it. It’s a critical component, as it’s part of your knowledge-base when you don't have perfect knowledge. Because if there is no perfect knowledge and you take away the uncertainty aspect, then you go into the guesswork.
The Relationship Between Knowing And Using Knowledge
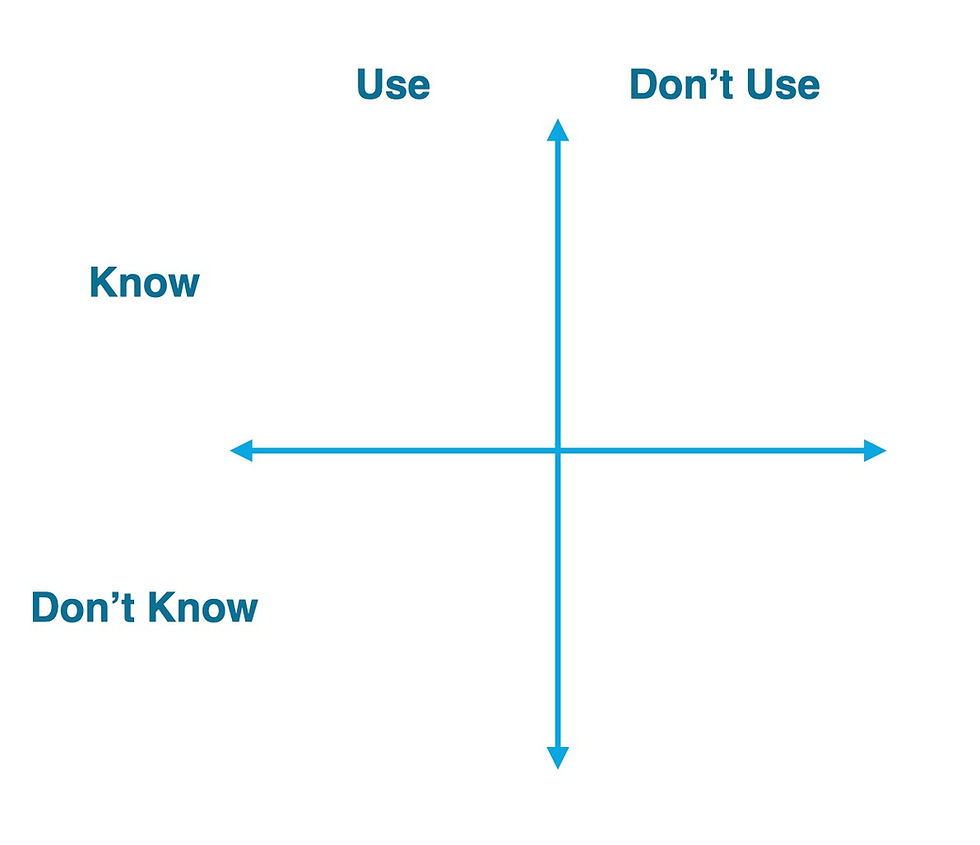
Another way to look at uncertainty is with a two by two, a favorite tool among analysts. This two by two demonstrates the relationship between knowing and usage of knowledge. You can either know something, or you don't know it. To that you can use it, or you can refrain from using it. By and large, this means that if you know something, and you use it, you create value. An example here is when you listen to the weather forecast, which says it might rain. To mitigate this risk when you're going out there, before you go out the door, you go into the closet, pick up an umbrella, and take it with you. That is value creation.
If you know something, but you don't use it, you are actually not creating value. An example of this is if you find a carton of milk in your refrigerator, and you look at the best before date, and it has passed by two days. Most people have a very good sense of smell, and most also know what sour milk smells like. Hence, in order to use your knowledge about sour milk, you would open the cart and smell it. And then depending on the smell, you would either pour it into a glass or pour it into the sink. However, there are people who don't trust their sense of smell, or they just don't use the knowledge they have, meaning that they just look at the date, sees that the date has passed by two days, and pours it into the sink. In that case, you're actually losing value by not using information.
If you don't use information that you don't know, that's actually a good thing. For example, letting a carpenter do some work that you're not qualified or knowledgeable enough to do. Instead of doing it yourself, risking a mishap, you instead let someone else use their knowledge and make the decisions that creates value for you.
Finally, you have the last quadrant, where you use information that you don’t have. This could be described as a person who says ”How hard can it be?” about driving a car, even though he/she actually can't drive. Nevertheless, they still attempt to drive it and will consequently very quickly run into something. This is not good decision making. Evidently, in the end, you need to use the knowledge you have to create value, as the value of information lies not in ownership, but in use.
With this theoretical background of uncertainty and value creation, you can know transfer this understanding to project or portfolio modeling. Because if you use point estimates without weighing in uncertainty, you’re actually leaving money on the table, as you’re not creating as much value as you could be. This because you're refraining from using information that you actually have.
Expressing Uncertainty
Before you even attempt to express uncertainty, you must listen to the experts, have them tell the story, and then figure out how to translate that story into a qualitative and quantitative model. After you have done that, the first way to describe an uncertainty is to use a probability distribution. In the chart below, you have a what's called a normal distribution, defined by a 90% interval. What this means is that if you are asked a question, let's say e.g the duration of a phase, the answer will be a different result every time you are asked. But, if you combine all those answers together, they're going to trend toward the average of this distribution, which in this case is seven and a half, in the middle of the range five to ten.

The normal distribution has tails on both ends, and being a 90% interval, this means that 5% of the time you are asked for a value, the answer is going to be something less than five, and 5% of the time, it's going to be something more than 10. So that's a way of expressing uncertainty in for example duration duration of an activity, the cost of something, or something else.
Another way of expressing uncertainty is a combined qualitative and quantitative insert, where you describe an event.
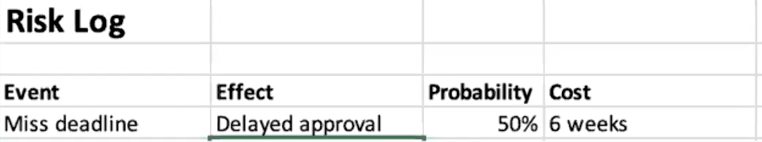
In the example above, you miss a deadline for a submission to a decision body. What you do then is to describe the effects should that event occur. In this case, the effect would be a delayed approval, as the decision body only meets once every six weeks. Here you can easily describe the cost, you can quantify the cost because if you just missed the slot, then next chance is going to be six weeks later. Thus, that’s going to be the cost to this risk. Then what you want to do is to estimate the probability that the event occurs, and in this case, the estimate is set to 50%. It’s important to assure that these estimates are not just guesses, but rather based on inputs from subject matter experts.
Subsequently, these are some of the ways of expressing the uncertainty. And with them, you can create models with a qualitative sequence flow, and describe the effects of decisions as you move from one activity to the next, or where you do things in parallel. At every stage you can incorporate uncertainty, better reflecting the uncertain reality which we live and operate in, so that you make a more informed decision.
Let’s do another example. Below you’ll see a model, in this case the model represents a launch date, that does not incorporate uncertainty in its calculations.
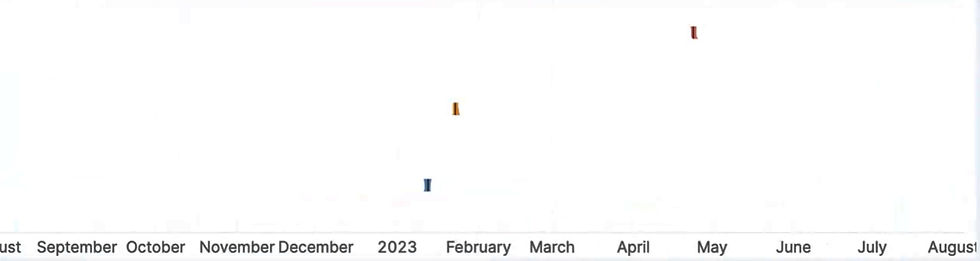
In the model there are three options, you can choose the top option, the middle option, or the bottom option. Obviously, looking at point values, the bottom option seems the best, as it will allow you to launch sooner than the other two. But if you look the full picture, i.e you include uncertainty to your model, you will get a range of outcomes.

What this model says is that the bottom alternative is best as an expected value, due to the fact that you launch earlier. But it also carries with it a risk that you will be even later than the middle option. Nevertheless, there's also a possibility that you will be even earlier. So in the end, you may end up making the same decision anyway, but the decision you made was augmented by the fact that you looked at the risk of being late versus the possibility the opportunity of actually being faster, and weighing those alternative routes against each other.
Conclusively, one can argue that there is value in uncertainty. If you use it, you can get better support for your decisions, and more data., as you understand both the expected but also the unexpected. With understanding uncertainty and by including it in your calculations, you can sort of practice the bad scenarios and the good scenario scenarios before they actually occur! This eventually boils down to a better ability to manage risks and opportunities, which is crucial if you want to have more confidence in your decisions to invest more money or resources.
Reference
Armour, G.Philip. (2010). The Five Order of Ignorance. COMMUNICATIONS OF THE ACM. Vol. 43, No. 10



Comments